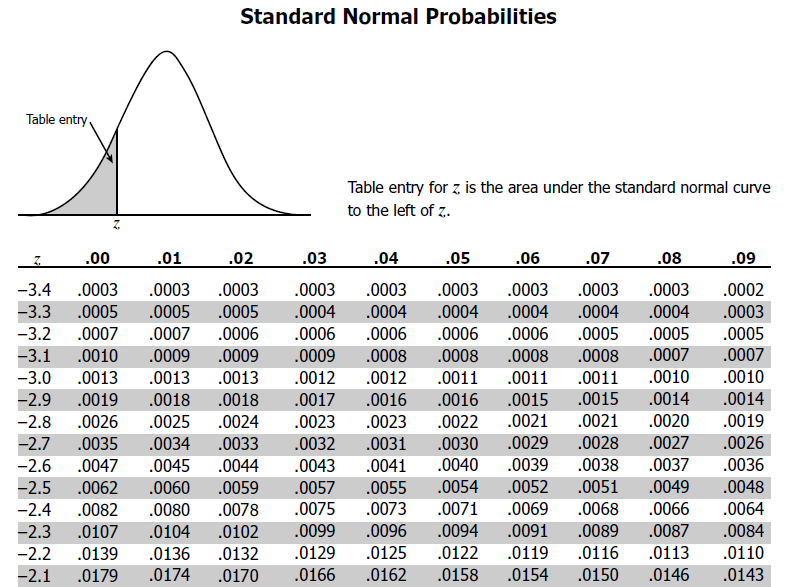
Say we're looking for the area left of -2.84. (Z-values with more accuracy need to be rounded to the hundredths in order to use this table.) To determine which z-value it's referring to, we look to the left to get the first two digits and above to the columns to get the hundredths value. That's the key - the values in the middle represent areas to the left of the corresponding z-value.
It's pretty overwhelming at first, but if you look at the picture at the top (take a minute and check it out), you can see that it is indicating the area to the left. You can download a printable copy of this table, or use the table in the back of a textbook. Before we start the section, you need a copy of the table. Finding Area under the Standard Normal Curve to the Leftīefore we look a few examples, we need to first see how the table works.
As we noted in Section 7.1, if the random variable X has a mean μ and standard deviation σ, then transforming X using the z-score creates a random variable with mean 0 and standard deviation 1! With that in mind, we just need to learn how to find areas under the standard normal curve, which can then be applied to any normally distributed random variable.